
$$ 0 = \mu \sin(\theta) - \cos(\theta) $$īut it is totally counter intuitive (and incorrect by experiment), that this point depends only on $\mu$ and not on the initial height $H$.

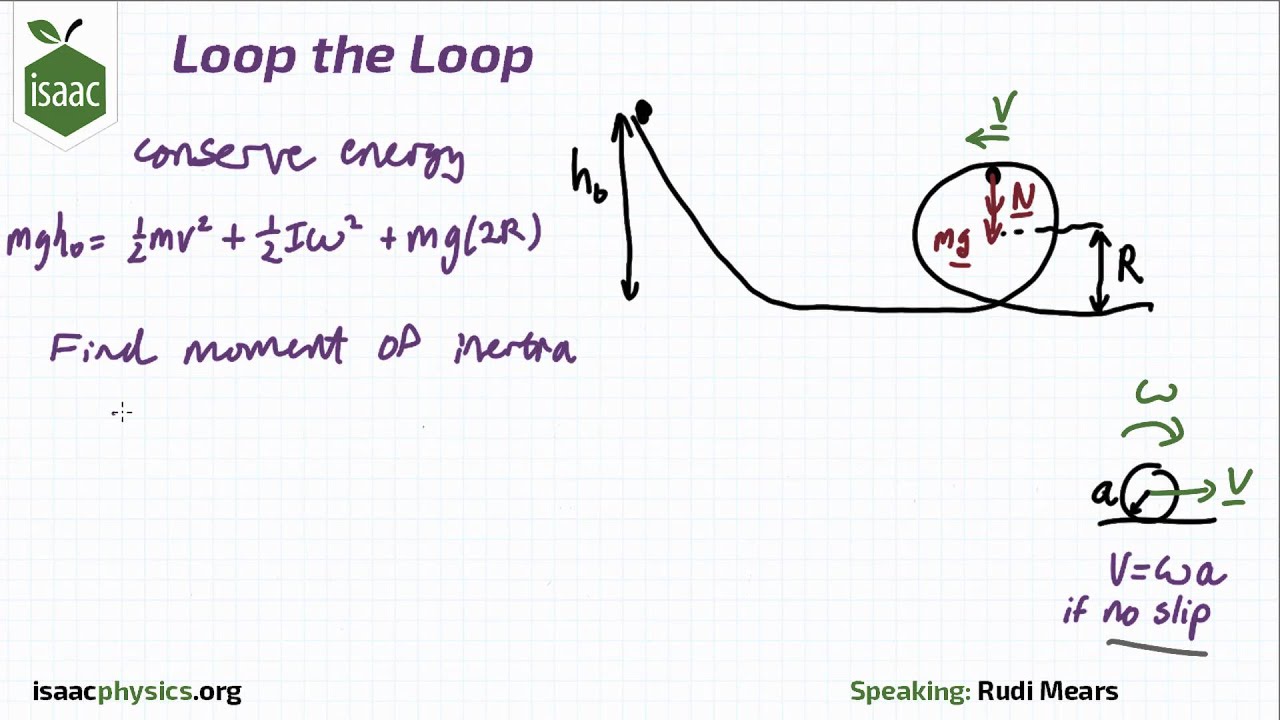
$$ mgH = \frac +gR(\mu\sin(\theta) -cos(\theta)) + \mu v(\theta)^2$$Īssuming the block doesn't make it, because it lost's its speed to friction, let's find $\theta$ for which $V(\theta) = 0$. Ignoring air resistance, but taking into account a friction coefficient of $\mu$ against the track, find the smallest $H$ for which the block will complete the loop.īy conservation of energy I have obtained the following equation: We drop a block from height $H$ that falls and goes around the loop.

The proportion of correlations that is higher than |.3| is 0.034 in this case.Let's consider a track that begins vertically becomes a 450 degree loop, and level off. 3īelow is the resulting histogram of the 5000 correlations. N 0.3) / nrun # look at the proportion of runs that have cor >. VK1SV Small circular loop calculator Enter loop radius in metres: Enter number of turns: Enter transmitter power in watts: Enter antenna impedance (. You want to find the absolute correlations >. Potential energy (PE) is the energy the object has due to its position. Work (W) is the energy given to the object by applying a force over a distance. Enter loop radius in metres: Enter number of turns: Enter transmitter power in watts: Enter antenna impedance (dominated by loop resistive loss) in ohms: Enter frequency in MHz: Enter loop inductance in uH: (Single turn loop inductance calculator here ) The wavelength in metres is: The loop area in square. Therefore, the minimum velocity the mouse needs to make it past the top of the loop without falling is: 0.25 km. In that calculations 1, which are based on the current loop model. The three types of energy that we will be considering are: Work, Potential Energy, and Kinetic Energy. m g H m v ( ) 2 2 + m g R ( 1 + sin ( )) + m R 0 ( g sin ( ) + v ( ) 2 R) d Where v ( ) the the block's speed at, and is an angle measured between the block's position (centred at loop center) and a horizontal line going left-wards. 3Department of Physics, Faculty of Science, University of Aksaray, Aksaray, Turkey. To use the vertical-loop velocity formula, you need to first convert the given velocity to units of meters per second (to match the meters per second squared of g ): Therefore: Finally, multiply by 2 to get the diameter and then. In this simple device a small sphere typically rolls down an incline and then continues around a circular track, which constitutes the loop. 2.2 C1:Circle There are two ways by which we can nd the minimal surface - (a) solve the equation of motion and (b) make use of conformal symmetry. Therefore, the minimum velocity the mouse needs to make it past the top of the loop without falling is: 0.25 km. Current, Part II A current loop in the x-y plane has a. 3 outside of the for loop.Įdit: One final correction is needed in the bracketing of the absolute function. Loop the Loop The loop the loop is an example of conservation of energy. In the next example, we shall see how we can use this trivial result to compute the Wilson loop for a circular contour. a) Calculate the magnitude and direction of the magnetic field at the center of the square loop. There is a small additional complication in that the track can loop around. One of the students realized the relevance of pendulum motion to understand a roller coasters loop: both situations involve the same physics concepts and students often forget the centripetal acceleration at the bottom, as documented for pendulum motion, e.g. This results in a vector of 5000 correlations which you can use to look at the proportion of correlations (divide by the number of runs, not the number of observations) that are higher than. The shape is determined by those equations where the value of t goes between. This equation becomes B 0nI /(2R) B 0 n I / ( 2 R) for a flat coil of n loops per length. You want new data for each run of the loop (otherwise you get the same correlation 5000 times) and you need to save the correlation each time the loop runs. By setting y 0 y 0 in Equation 12.16, we obtain the magnetic field at the center of the loop: B 0I 2R j.
Loop the loop physics calculator code#
You just had to shift some code outside and inside the for loop.


 0 kommentar(er)
0 kommentar(er)
